Today’s SAT question of the day is a fun math question that reviews some geometry basics. You’ll definitely need those basics for your test, so let’s give this one a try.
We are told that the radius of circle O is 5 and that the area of the shaded region is 20pi. X is what we are seeking.
Since the area of a circle is pi * the radius squared, we know that the whole circle’s area is 25pi. Let’s also remember that there are 360 degrees in a circle. Continue reading
Today’s SAT Question of the day is a quick and easy math problem. The test makers would like to know how many non-overlapping squares with side length 3 can fit inside a square with side length 6. My favorite way to solve this is with some quick sketches:
Today’s ACT question is about the area of a trapezoid – they were nice enough to give us that formula right in the problem, so it’s a piece of cake! Careful substitution cake with algebra frosting.
The formula they give us is:
a = (1/2)*h*(b1 + b2) (h is the altitude, b1 and b2 are the parallel bases)
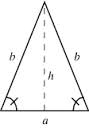
Today’s ACT question of the day involves a triangle with two equal sides. Triangles with two equal sides (and, therefore, two equal angles) are isosceles triangles. This is a key observation to make before taking on this problem.
We have an isosceles triangle with only a base (length of 10). The height has been drawn in, bisecting the third angle and meeting the base at a right angle, but we don’t know its length. It would be nice if we did, right? Area of a triangle = 1/2 • base • height.
If we knew any of the angle measures, maybe we could luck out and find a 45-45-90 or 30-60-90 triangle somewhere in the diagram. We could also be sharp and recall one of our Pythagorean triplets: 3, 4, 5. Unfortunately, we don’t have any facts to support those guesses. We do have answer choices that work if you make those spurious assumptions, so be careful! Don’t make things up just to make the problem seem easier.
We could try to use trigonometry creatively to derive the hypotenuse, then work backward to find the height. Unfortunately, cos90 = 0, so that ends right there. If we knew the angle at the top of the triangle, we could use the sin of that angle • half the square of the length of one of the equal sides to find the area (works for all isosceles triangles)…but we have neither measure. What now?
Image copyright The College Board
Today’s official SAT question of the day is about a shaded circle inscribed in a semicircle. Given only the radius of the semicircle, we are asked to find the area of the shaded circle.
How to start:
From the diagram and the given information, we must notice that the circle’s diameter is also a radius of the semicircle; this segment is labeled CD.